A new MOX report entitled “A roughness penalty approach to estimate densities over two-dimensional manifolds” by Arnone, A.; Ferraccioli, F.; Pigolotti, C.; Sangalli, L.M. has appeared in the MOX Report Collection.
The report can be donwloaded at the following link:
https://www.mate.polimi.it/biblioteca/add/qmox/41/2022.pdf
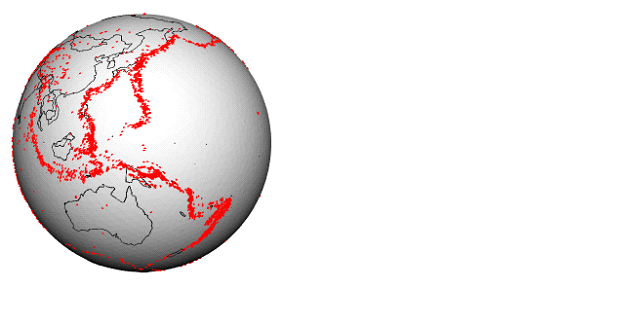
Abstract: An innovative nonparametric method for density estimation over general two-dimensional Riemannian manifolds is proposed. The method follows a functional data analysis approach, combining maximum likelihood estimation with a roughness penalty that involves a differential operator appropriately defined over the manifold domain, thus controlling the smoothness of the estimate. The proposed method can accurately handle point pattern data over complicated curved domains. Moreover, it is able to capture complex multimodal signals, with strongly localized and highly skewed modes, with varying directions and intensity of anisotropy. The estimation procedure exploits a discretization in finite element bases, enabling great flexibility on the spatial domain. The method is tested through simulation studies, showing the strengths of the proposed approach. Finally, the density estimation method is illustrated with an application to the distribution of earth! quakes in the world.