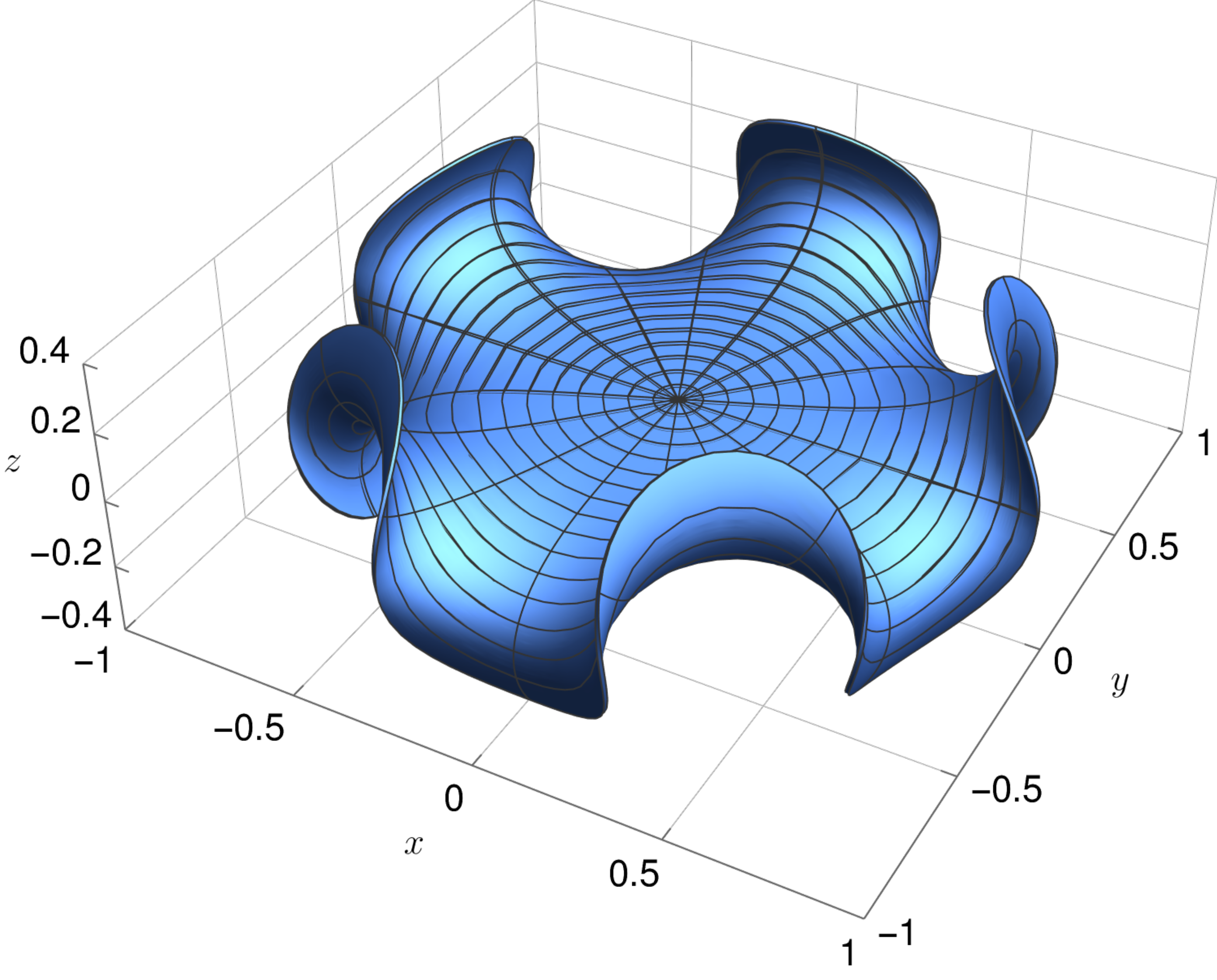
A new MOX report entitled “The Föppl–von Kármán equations of elastic plates with initial stress” by Ciarletta, P.; Pozzi, G.; Riccobelli, D. has appeared in the MOX Report Collection.
The report can be donwloaded at the following link:
https://www.mate.polimi.it/biblioteca/add/qmox/28/2022.pdf
Abstract: Initially stressed plates are widely used in modern fabrication techniques, such as additive manufacturing and UV lithography, for their tunable morphology by application of external stimuli. In this work, we propose a formal asymptotic derivation of the Föppl–von Kármán equations for an elastic plate with initial stresses, using the constitutive theory of nonlinear elastic solids with initial stresses under the assumptions of incompressibility and material isotropy. Compared to existing works, our approach allows to determine the morphological transitions of the elastic plate without prescribing the underlying target metric of the unstressed state of the elastic body. We explicitly solve the derived FvK equations in some physical problems of engineering interest, discussing how the initial stress distribution drives the emergence of spontaneous curvatures within the deformed plate. The proposed mathematical framework can be used to tailor shape on demand, with applications in several engineering fields ranging from soft robotics to 4D printing.